
- #Arithmetic and geometric sequences and series how to
- #Arithmetic and geometric sequences and series series
Textbook content produced by OpenStax is licensed under a Creative Commons Attribution License.

We recommend using aĪuthors: Lynn Marecek, Andrea Honeycutt Mathis Use the information below to generate a citation. Maths Algebra Revise Test 1 2 3 4 5 6 Geometric sequences In a (geometric) sequence, the term to term rule is to multiply or divide by the same value. Then you must include on every digital page view the following attribution: If you are redistributing all or part of this book in a digital format, Then you must include on every physical page the following attribution: If you are redistributing all or part of this book in a print format, Want to cite, share, or modify this book? This book uses the The twelfth term of the sequence is 0, a 12 = 0. Ī 12 = 22 + ( 12 − 1 ) ( −2 ) a 12 = 22 + ( 12 − 1 ) ( −2 )Ī 12 = 22 + ( 11 ) ( −2 ) a 12 = 22 + ( 11 ) ( −2 ) Practice identifying both of these sequences by watching this tutorial Keywords: sequence arithmetic sequence geometric sequence common ratio common.
#Arithmetic and geometric sequences and series how to
Then we will investigate different sequences and figure out if they are Arithmetic or Geometric, by either subtracting or dividing adjacent terms, and also learn how to write each of these sequences as a Recursive Formula.Īnd lastly, we will look at the famous Fibonacci Sequence, as it is one of the most classic examples of a Recursive Formula.To first find the first term, a 1, a 1, use theįormula with a 7 = 10, n = 7, and d = −2.
#Arithmetic and geometric sequences and series series
An infinite geometric series is a series of the form. Arithmetic and geometric sequence and series Study Development Worksheet Answers 1. I like how Purple Math so eloquently puts it: if you subtract (i.e., find the difference) of two successive terms, you’ll always get a common value, and if you divide (i.e., take the ratio) of two successive terms, you’ll always get a common value. Similar to an arithmetic sequence, a geometric sequence is determined completely by the first. Geometric: ¾, 3, 12, 48, 192, a 1 ¾ common ratio 4 Recursive Definition (Formula) of a Sequence In order to describe a sequence to someone, we simply must tell them where to start, and then how. Then, we either subtract or divide these two adjacent terms and viola we have our common difference or common ratio.Īnd it’s this very process that gives us the names “difference” and “ratio”. Geometric Sequences Geometric Sequences are built by repeatedly multiplying the same number (called the common ratio) to the first term a 1. And adjacent terms, or successive terms, are just two terms in the sequence that come one right after the other. Well, all we have to do is look at two adjacent terms. A geometric sequence has a constant ratio between each pair of consecutive terms. This is similar to the linear functions that have the form y m x + b. An arithmetic sequence has a constant difference between each consecutive pair of terms. FROM PATTERNS TO GENERALIZATIONS: SEQUENCES, SERIES AND PROOF. Two common types of mathematical sequences are arithmetic sequences and geometric sequences.
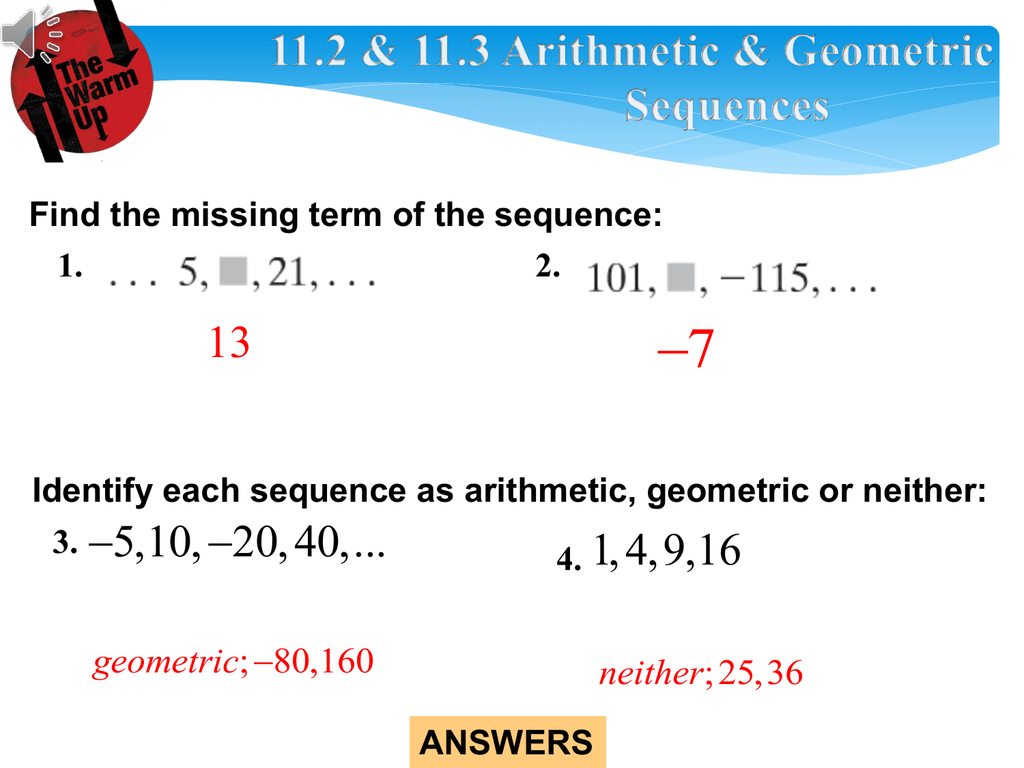
It’s going to be very important for us to be able to find the Common Difference and/or the Common Ratio. Arithmetic and geometric sequences and series. Two common types of mathematical sequences are arithmetic sequences and geometric sequences. The figure below shows all sequences and series formulas. In a harmonic sequence, the reciprocals of its terms are in an arithmetic sequence. In a geometric sequence, there is a common ratio between consecutive terms.

Comparing Arithmetic and Geometric Sequences In an arithmetic sequence, there is a common difference between two subsequent terms.


 0 kommentar(er)
0 kommentar(er)
